Visit wwwdoucehousecom for more videos like this In this video, I explain in more detail about the special right triangle I also solve 4 diffeYou can also recognize a 30°60°90° triangle by the angles As long as you know that one of the angles in the rightangle triangle is either 30° or 60° then it must be a 30°60°90° special right triangle A right triangle with a 30° angle or 60° angle must be a 30°60°90° special right triangle Side1 Side2 Hypotenuse = x x√3 2x Example 1A triangle is a right triangle where the three interior angles measure 30° 30 °, 60° 60 °, and 90° 90 ° Right triangles with interior angles are known as special right triangles Special triangles in geometry because of the powerful relationships that unfold when studying their angles and sides
A Full Guide To The 30 60 90 Triangle With Formulas And Examples Owlcation
Special triangles 30 60 90 chart
Special triangles 30 60 90 chart-Special Triangles Isosceles and Calculator This calculator performs either of 2 items 1) If you are given a right triangle, the calculator will determine the missing 2 sides Enter the side that is known After this, press Solve Triangle 2) In addition, the calculator will allow you to same as Step 1 with a right triangle The most frequently studied right triangles the special right triangles are the 30 60 90 triangles followed by the 45 45 90 triangles 14 the length of one side of an equilateral triangle is 6meters Find the lengths of the other sides




Special Right Triangles In Geometry 45 45 90 And 30 60 90 Youtube
PreCalculus Course https//wwwyoutubecom/c/MrHelpfulNotHurtful/playlists?view=50&sort=dd&shelf_id=3How do we find the unknown sides of special right triaUsing what we know about triangles to solve what at first seems to be a challenging problem Created by Sal Khan Special right triangles Special right triangles proof (part 1) Special right triangles proof (part 2) Practice Special right triangles triangle example problem This is the currently selected itemA special kind of triangle A right triangle (literally pronounced "thirty sixty ninety") is a special type of right triangle where the three angles measure 30 degrees, 60 degrees, and 90 degrees The triangle is significant because the sides exist in an easytoremember ratio 1sqrt (3)2 That is to say, the hypotenuse is twice as long as the shorter leg, and the longer leg is the
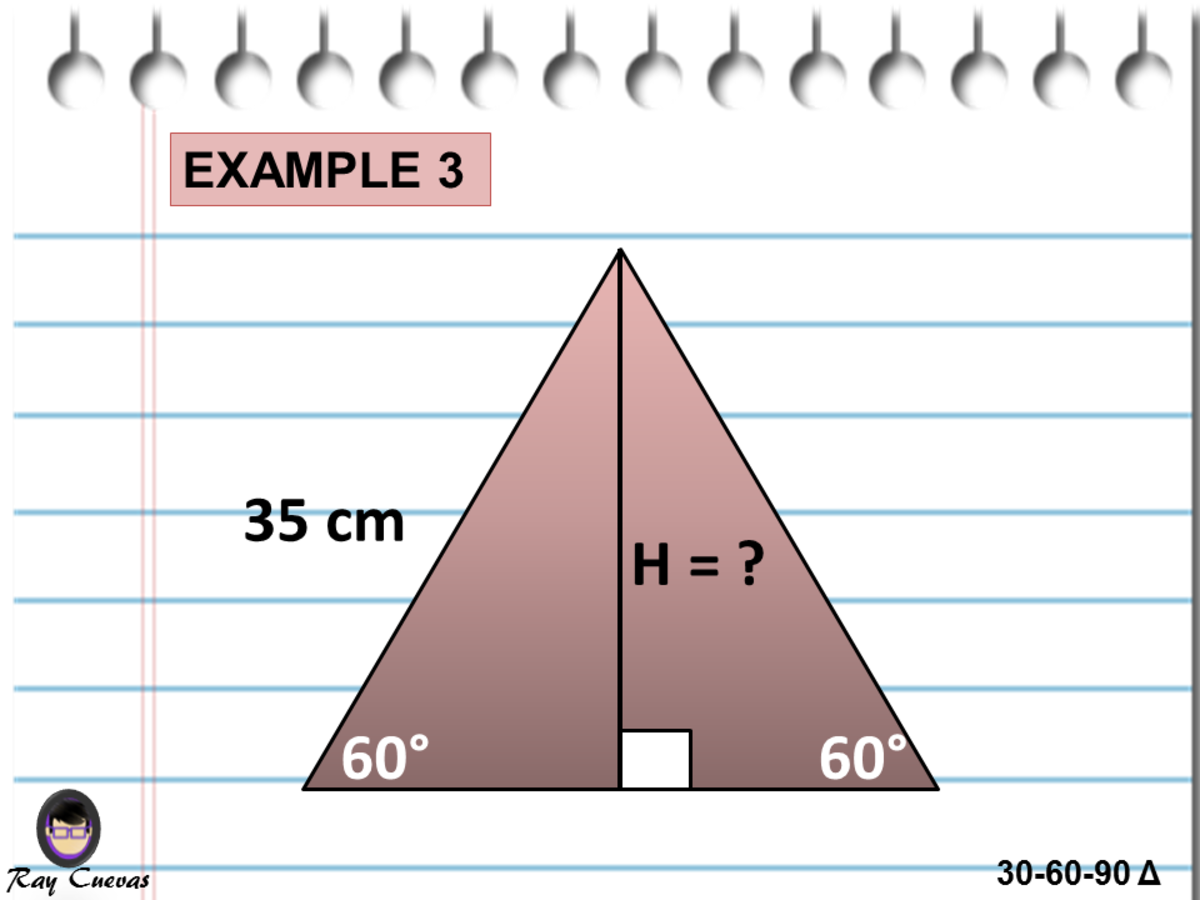
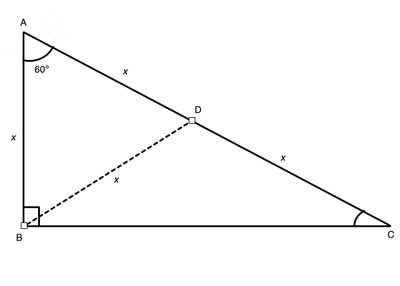
Triangles Theorem 2 In a triangle whose angles measure 30 0, 60 0, and 90 , the hypotenuse has a length 0 equal to twice the length of the shorter leg, and the length of the longer leg is the product of 3 And the length of the shorter leg The ratio of the sides of a triangle are x x 3 2 x Note The short leg is always opposite the 30 ° angle!It has the shape of an equilateral triangle with a side length of 2 feet If the altitude of the triangular sign is drawn, you split the Yield sign in half vertically, creating two 30°60°90° right triangles, as shown to the right For now, we'll focus on the right triangle on the right sideIn any triangle, you see the following The shortest leg is across from the 30degree angle, the length of the hypotenuse is always double the length of the shortest leg, and you can find the length of the long leg by multiplying the short leg by the square root of 3
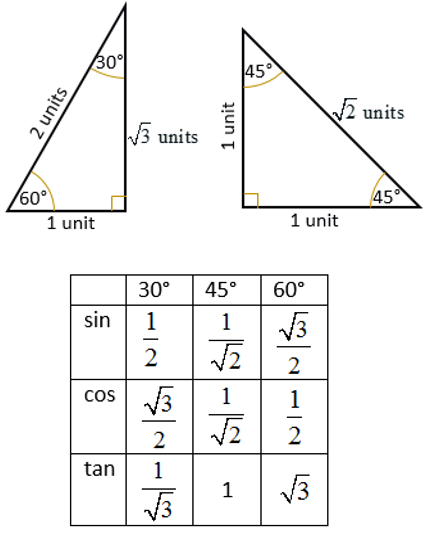
Use the Pythagorean theorem to discover patterns in 30°60°90° and 45°45°90° triangles This is often how triangles appear on standardized tests—as a right triangle with an angle measure of 30º or 60º and you are left to figure out that it's Not only that, the right angle of a right triangle is always the largest angle—using property 1 again, the other two angles will have to add up to 90º, meaning each of them can't beThe following special angles chart show how to derive the trig ratios of 30°, 45° and 60° from the and special triangles Scroll down the page if you need more examples and explanations on how to derive and use the trig ratios of special angles Trigonometric Function Values Of Special Angles How to derive the trigonometric function values of 30, 45 and 60



Precalculus Notes Trig 3




A Full Guide To The 30 60 90 Triangle With Formulas And Examples Owlcation
A triangle is a special right triangle (a right triangle being any triangle that contains a 90 degree angle) that always has degree angles of 30 degrees, 60 degrees, and 90 degrees Because it is a special triangle, it also has side length values which are always in a consistent relationship with one another 30 60 90 triangle sides If we know the shorter leg length a, we can find out that b = a√3 c = 2a If the longer leg length b is the one parameter given, then a = b√3/3 c = 2b√3/3 For hypotenuse c known, the legs formulas look as follows a = c/2 b = c√3/2 Or simply type your given values and the 30 60 90 triangle calculator will do the rest!Special Right Triangles You need to have separate sections for 45/45/90 special triangle and also, 30/60/90 special triangle Also explain if 30/60/90 and 60/30/90 triangles are same or different and why Explain how to use it to find the unknown side of another special right triangles and why you can do it Hint if two triangles are similar




Special Right Triangles 30 60 90 And 45 45 90 Triangles Youtube




Special Right Triangle 30 60 90 Mathbitsnotebook Geo Ccss Math
Special Right Triangles Although all right triangles have special features – trigonometric functions and the Pythagorean theorem The most frequently studied right triangles, the special right triangles, are the 30, 60, 90 Triangles followed by the 45, 45, 90 triangles



The Easy Guide To The 30 60 90 Triangle



1



30 60 90 Triangle Geometry Help



Free Special Right Triangles 30 60 90 Foldable By Mrs Newells Math



Trigonometric Ratios Of Special Angles 0 30 45 60 90 Video Lessons Examples And Solutions




A Full Guide To The 30 60 90 Triangle With Formulas And Examples Owlcation




30 60 90 Right Triangles Ck 12 Foundation
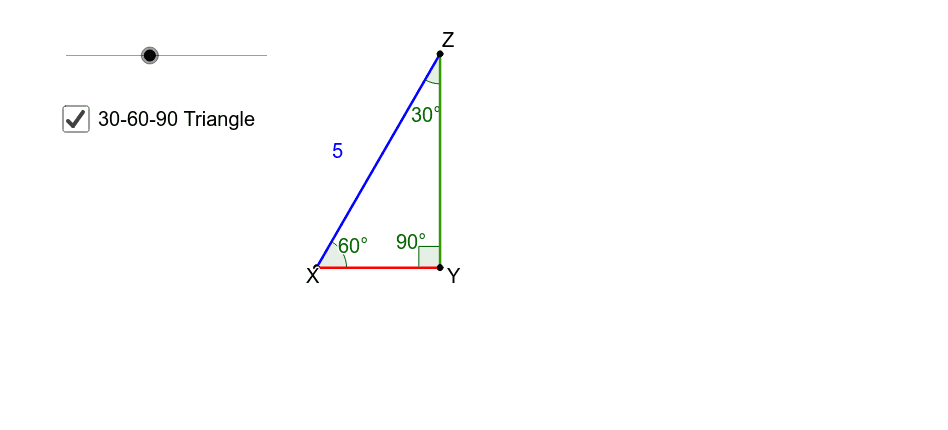



Special Right Triangles 30 60 90 Geogebra




Learn About The 30 60 90 Triangle Caddell Prep Online




Mrs Newell S Math Better Questions Special Right Triangles
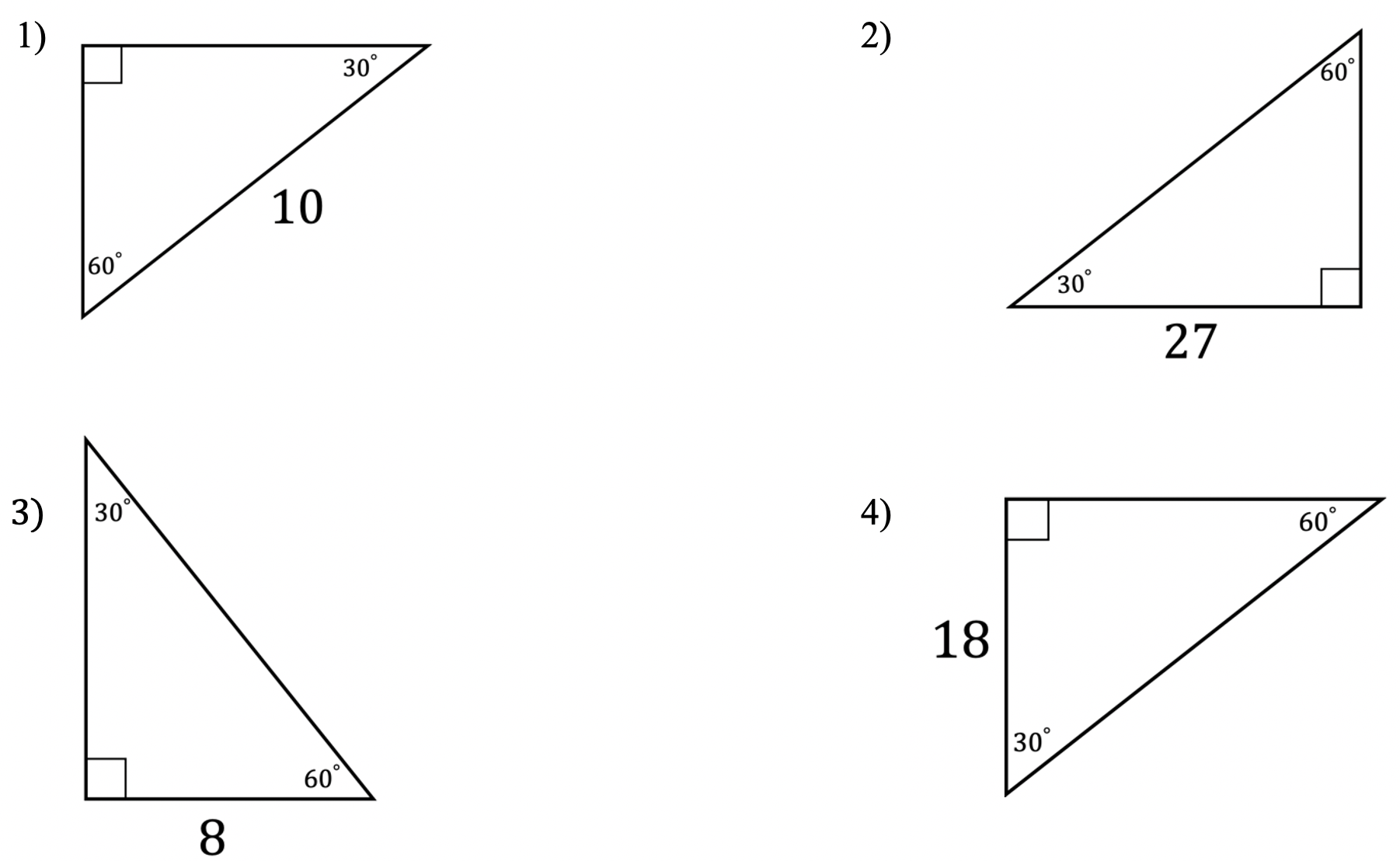



30 60 90 Special Triangles Geometry Mathsux 2



Discovering Special Right Triangles 30 60 90 Triangles Made With Medians Of Equilateral Triangles Activity Builder By Desmos
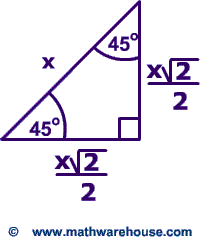



Special Right Triangles Formulas 30 60 90 And 45 45 90 Special Right Triangles Examples Pictures And Practice Problems




30 60 90 Triangle Theorem Ratio Formula Video




30 60 90 Triangle Explanation Examples




Unit Circle Chart
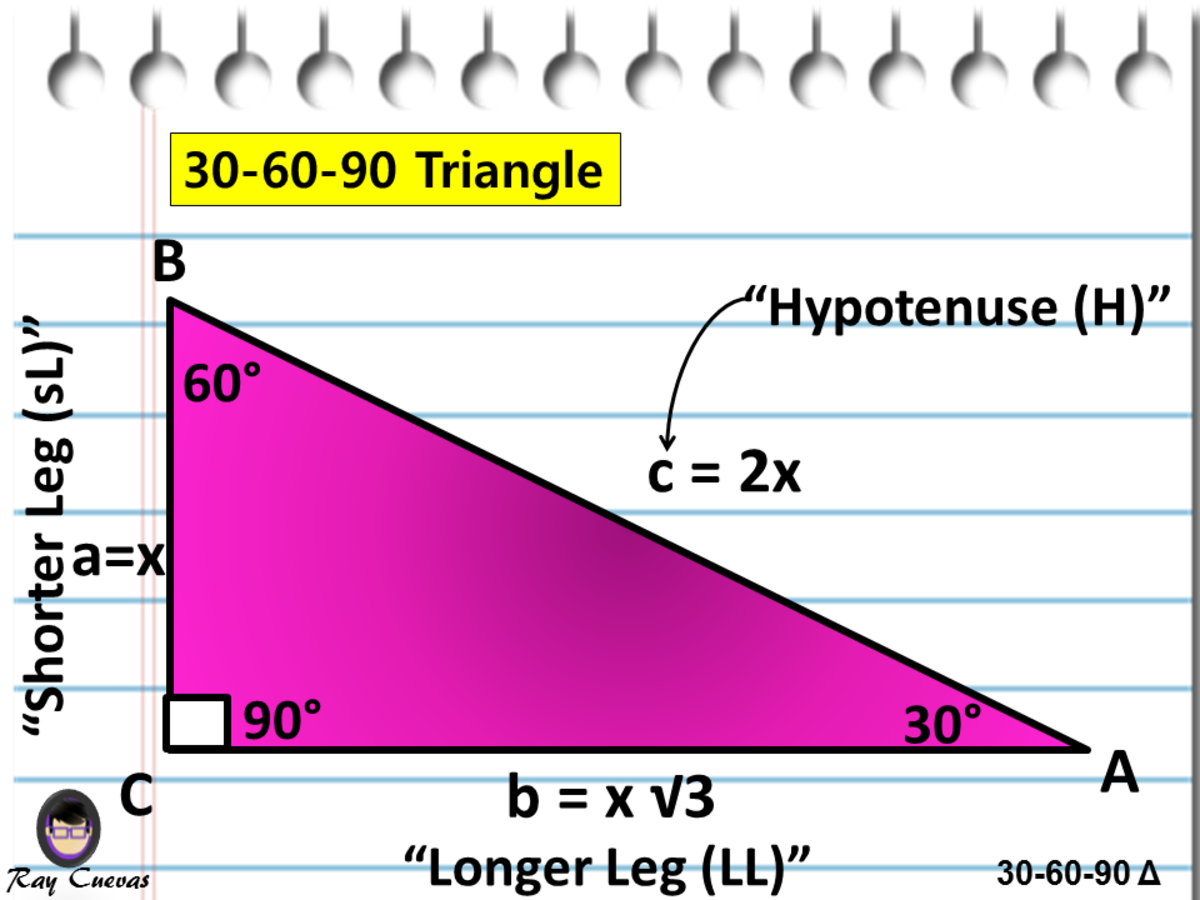



A Full Guide To The 30 60 90 Triangle With Formulas And Examples Owlcation




The Complete Guide To The 30 60 90 Triangle



3
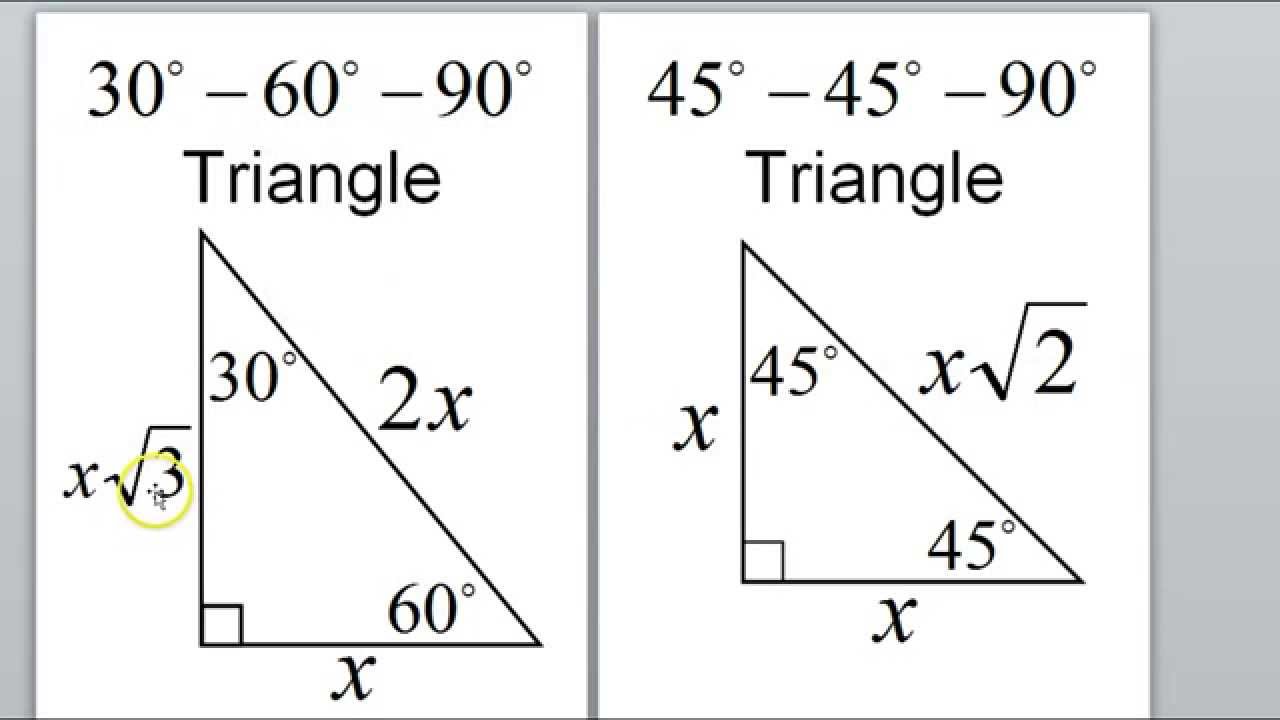



Day 1 Hw Special Right Triangles 45 45 90 30 60 90 Youtube




30 60 90 Triangle Explanation Examples




30 60 90 Triangle Calculator Formula Rules




Special Right Triangles Interactive Notebook Page Math Methods Teaching Geometry Teaching Math




Trig Values For Paper 1 Triangle Method Gcse
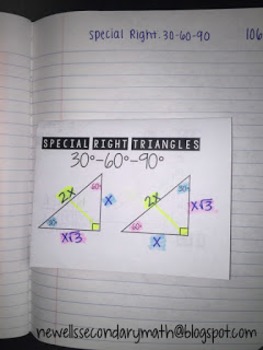



Free Special Right Triangles 30 60 90 Foldable By Mrs Newells Math




30 60 90 Right Triangles Solutions Examples Videos




Complex Special Triangles 45 45 90 30 60 90 Similar Triangles Geometry Trig Youtube
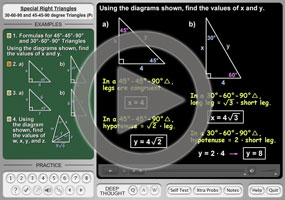



Special Angle Values 30 60 90 And 45 45 90 Triangles Purplemath




Identifying The 30 60 90 Degree Triangle Dummies
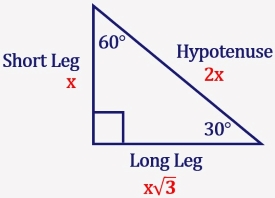



Special Right Triangle 30 60 90 Mathondemand Com
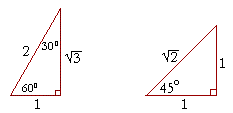



The 30 60 90 Triangle Topics In Trigonometry




Mrs Newell S Math Better Questions Special Right Triangles




How To Use The Special Right Triangle 30 60 90 Studypug




30 60 90 Triangle Explanation Examples




30 60 90 Special Triangles Geometry Mathsux 2




Trig Without A Calculator Mrarnold
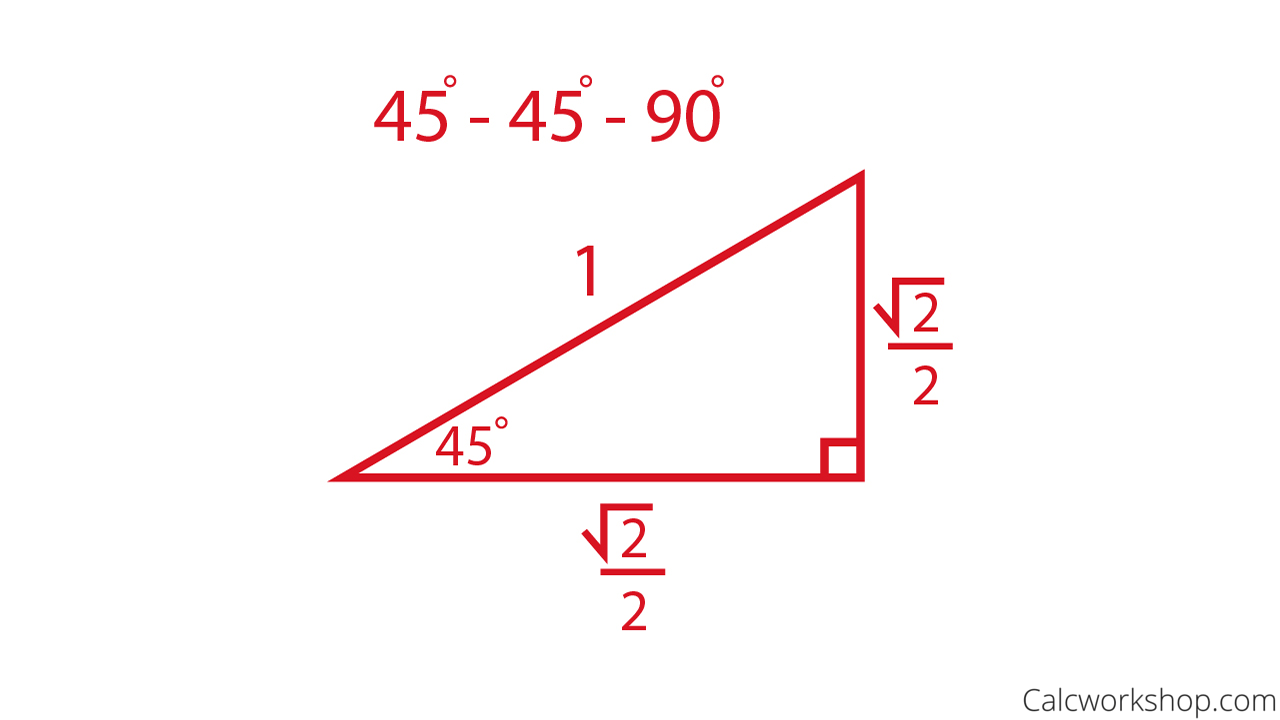



Unit Circle W Everything Charts Worksheets 35 Examples




Special Right Triangles Anchor Chart Math Interactive Notebook Fractions Anchor Chart Math Interactive



Precalculus Notes Trig 3
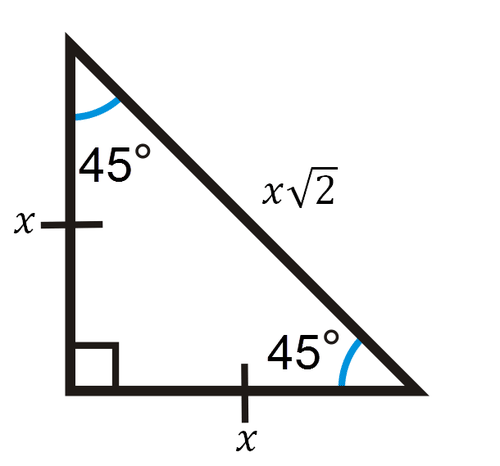



Special Right Triangles Ck 12 Foundation
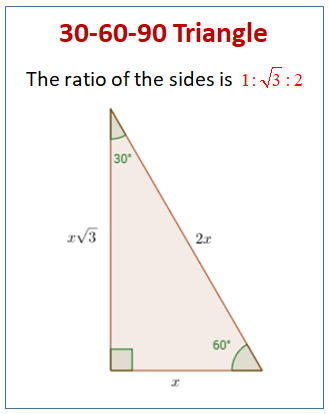



30 60 90 Special Right Triangles Videos Worksheets Examples Solutions




30 60 90 Triangle Calculator Formula Rules



The Easy Guide To The 30 60 90 Triangle
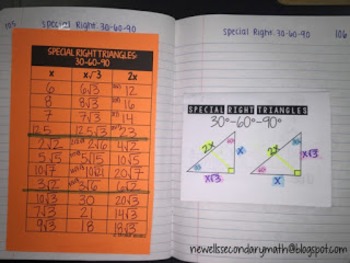



Free Special Right Triangles 30 60 90 Foldable By Mrs Newells Math




Special Right Triangle Wikipedia



The Easy Guide To The 30 60 90 Triangle




30 60 90 Triangle Theorem Ratio Formula Video
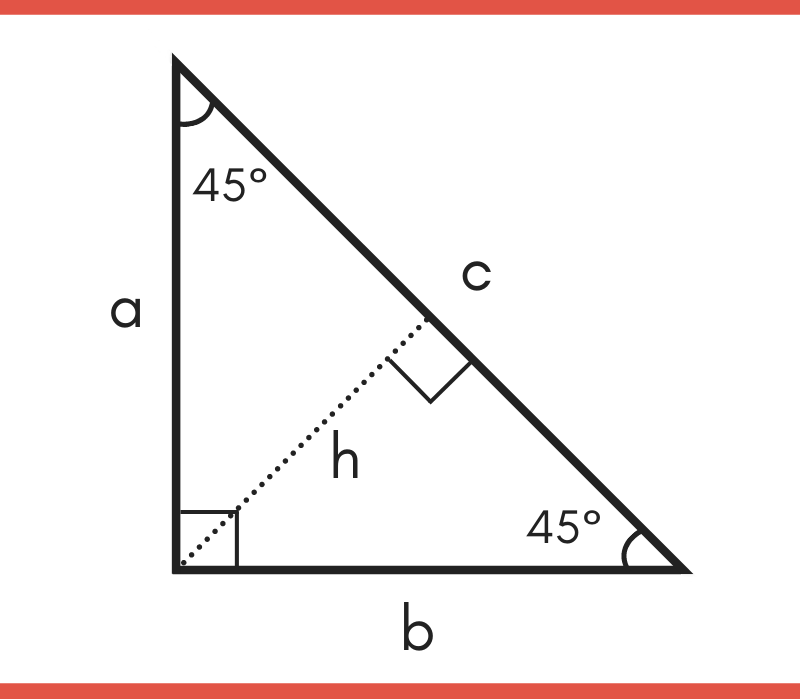



45 45 90 Special Right Triangle Calculator Inch Calculator



1



30 60 90 Triangle Rules
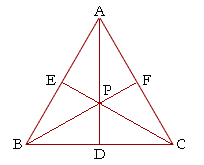



The 30 60 90 Triangle Topics In Trigonometry
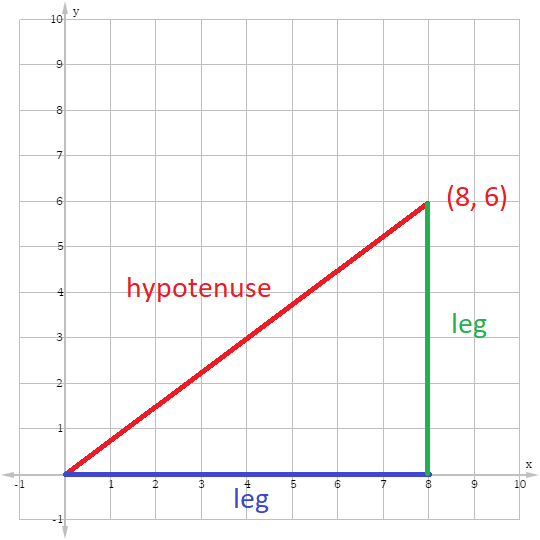



30 60 90 Triangle Formulas Rules And Sides Science Trends
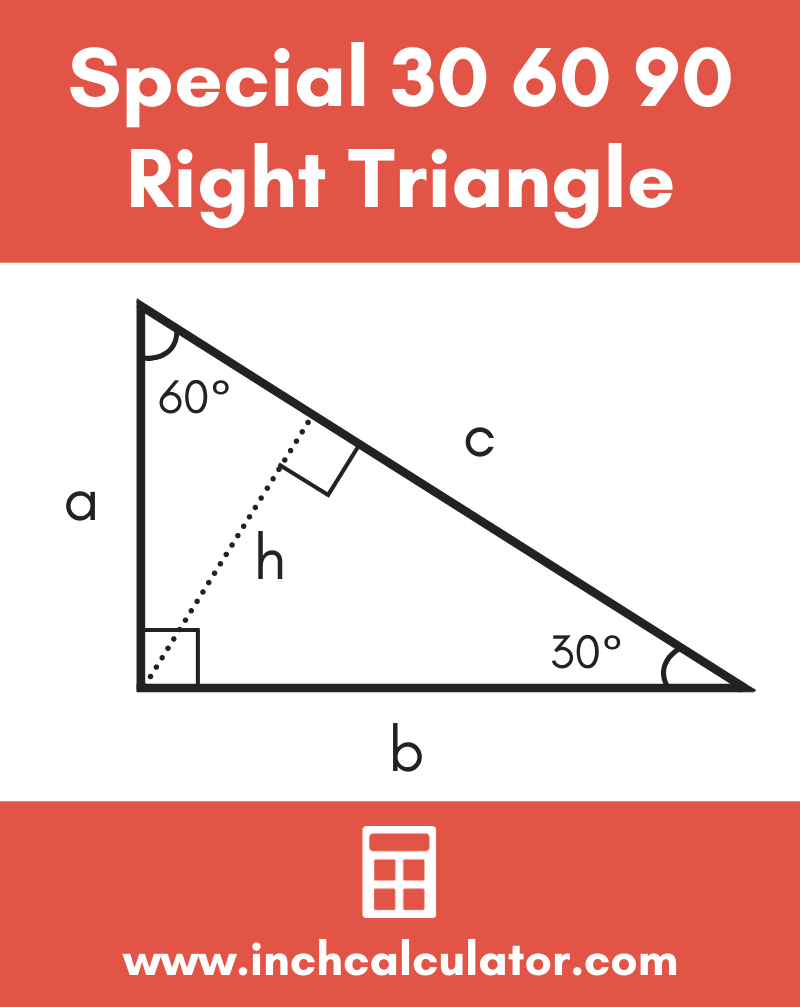



30 60 90 Special Right Triangle Calculator Inch Calculator




Mrs Newell S Math Better Questions Special Right Triangles




30 60 90 Triangle Diagram Quizlet



Answer In Geometry For Alden



45 45 90 And 30 60 90 Triangles Zona Land Education



Special Right Triangles Teacher Guide




Special Right Triangles In Geometry 45 45 90 And 30 60 90 Youtube
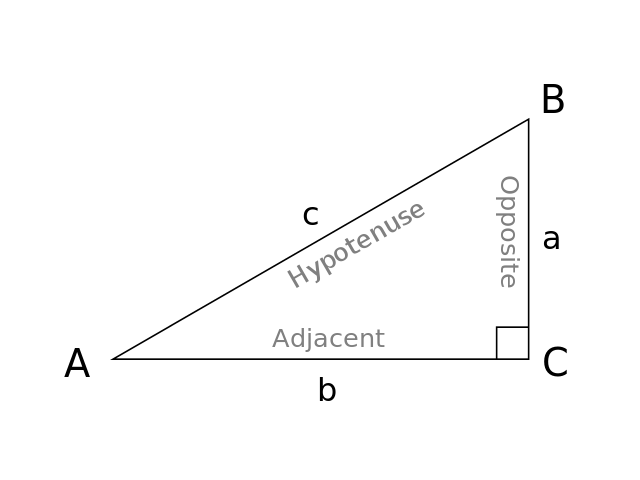



30 60 90 Triangle Formulas Rules And Sides Science Trends
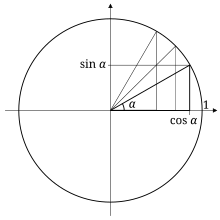



Special Right Triangle Wikipedia




A Quick Guide To The 30 60 90 Degree Triangle Dummies
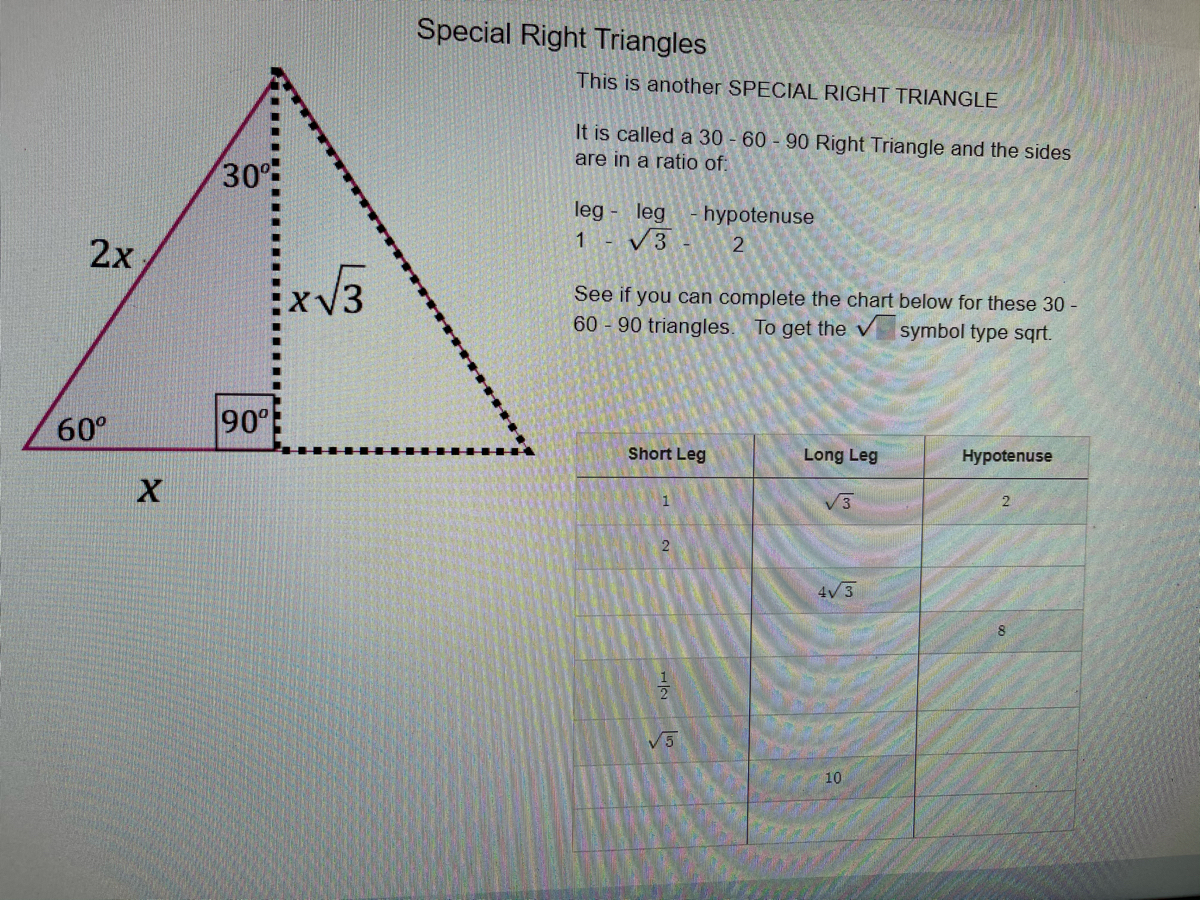



Answered Special Right Triangles This Is Another Bartleby
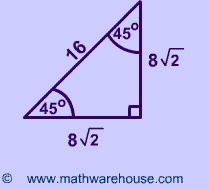



Special Right Triangles Formulas 30 60 90 And 45 45 90 Special Right Triangles Examples Pictures And Practice Problems




Trigonometry Special Triangles Youtube



1




30 60 90 Triangle Theorem Ratio Formula Video
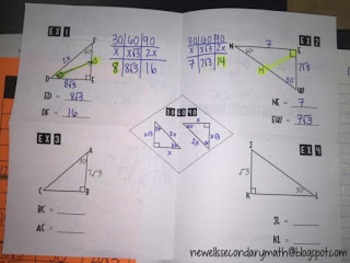



Free Special Right Triangles 30 60 90 Foldable By Mrs Newells Math



The Easy Guide To The 30 60 90 Triangle




Section 60 90 Triangles Ppt Download




How To Work With 30 60 90 Degree Triangles Education Is Around




Day 1 Hw Special Right Triangles 45 45 90 30 60 90 Youtube Right Triangle Trigonometry Worksheets Triangle Worksheet
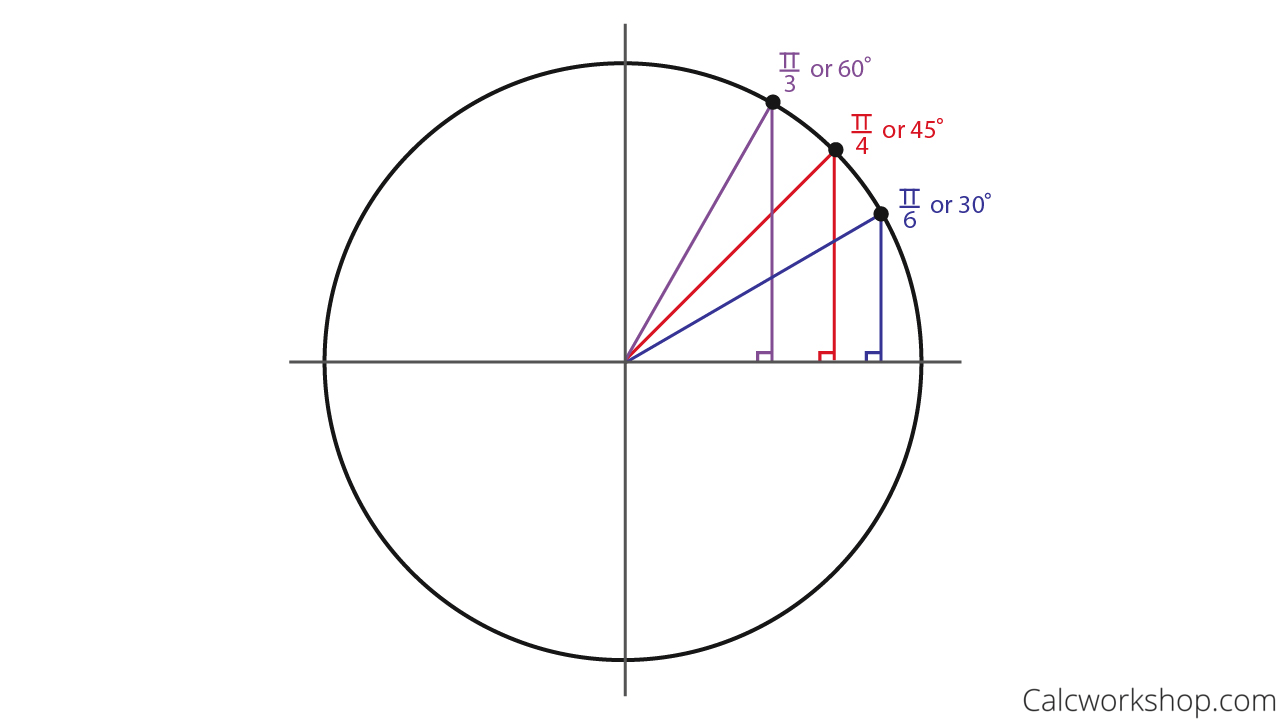



Unit Circle W Everything Charts Worksheets 35 Examples




30 60 90 Triangle Theorem Ratio Formula Video




30 60 90 Right Triangle Side Ratios Expii
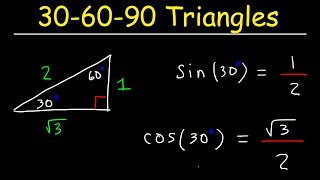



30 60 90 Triangles Special Right Triangle Trigonometry Youtube




Properties Of 3 4 5 Triangles Definition And Uses Video Lesson Transcript Study Com




A Full Guide To The 30 60 90 Triangle With Formulas And Examples Owlcation




File 30 60 90 Triangle Svg Wikimedia Commons Math Facts Simple Math Math Tricks




Solved If The Shortest Leg In The Following 30 60 90 Chegg Com




Special Right Triangles Fully Explained W 19 Examples




30 60 90 Special Right Triangle Calculator Inch Calculator
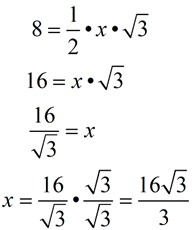



Special Right Triangle 30 60 90 Mathbitsnotebook Geo Ccss Math




5 30 60 90 Triangles Geometry15a




Special Right Triangles Review Article Khan Academy




30 60 90 Triangle Definition Theorem Formula Examples
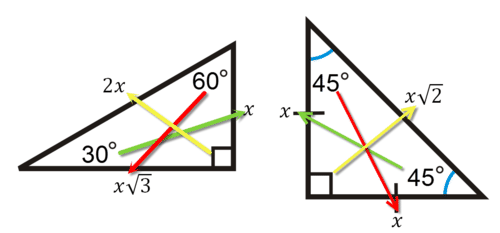



Special Right Triangles Ck 12 Foundation




30 60 90 Right Triangles Free Math Help




Special Right Triangles Middle School Geometry Anchor Charts Are In The Work Middle School Geometry Geometry Anchor Chart Middle School Anchor Charts




Special Right Triangles Video Lessons Examples And Solutions




Mrs Newell S Math Better Questions Special Right Triangles




30 60 90 Triangle Theorem Ratio Formula Video



Biomath Trigonometric Functions
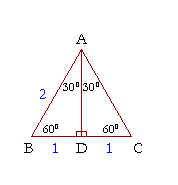



30 60 90 Right Triangle Side Ratios Expii
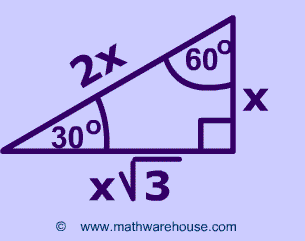



Special Right Triangles Formulas 30 60 90 And 45 45 90 Special Right Triangles Examples Pictures And Practice Problems
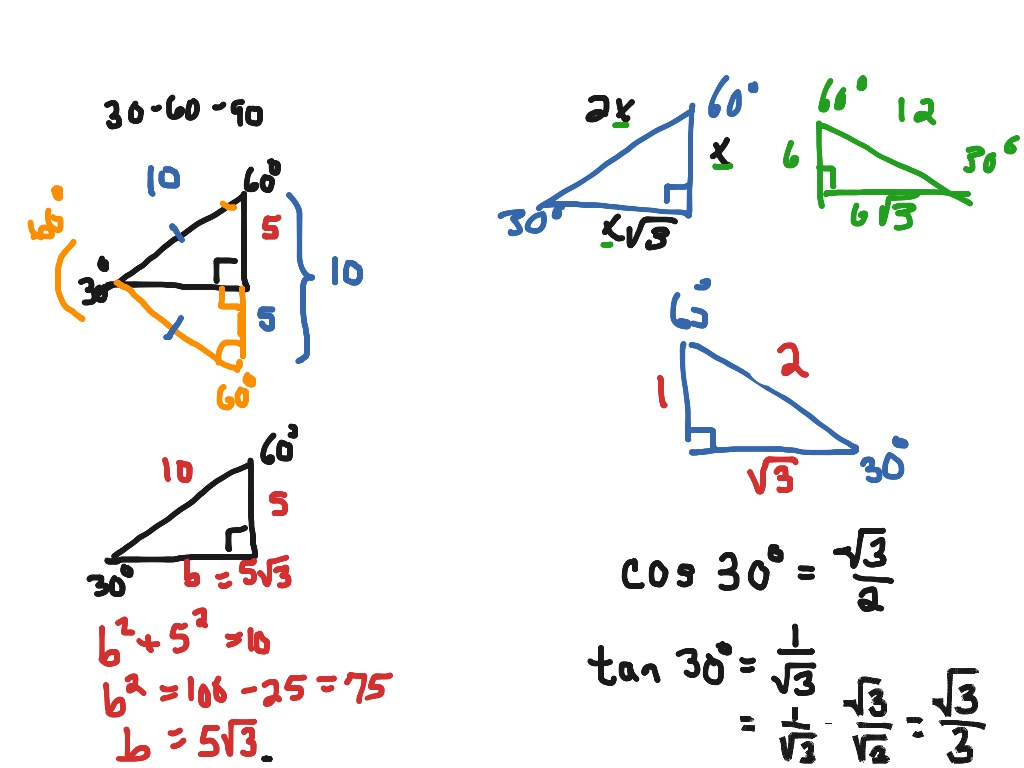



30 60 90 Right Triangles Math Trigonometry Trig Triangles Right Triangles Showme




30 60 90 Triangle Theorem Properties Formula Video Lesson Transcript Study Com




The Complete Guide To The 30 60 90 Triangle




30 60 90 Right Triangles Ck 12 Foundation




Mrs Newell S Math Better Questions Special Right Triangles



0 件のコメント:
コメントを投稿